Total Internal Reflection (TIR)

It is the process of reflection of light into a denser medium from an interface of the denser medium and a rarer medium.

Essential conditions for TIR : There are two essential conditions for the process to occur.

(i) Light should travel from denser medium to rarer medium

(ii) Angle of incidence in denser medium should be greater than the critical angle for the pair of media in contact.

Critical Angle : Critical angle for a pair of media in contact is the angle of incidence in the denser medium corresponding to which angle of refraction in the rarer medium is 90°. It is denoted by C and its value depend upon the nature of media in contact.

Let we have an interface XY separating a rarer medium (Air) and a denser medium (water). Let medium (a) is air and medium (b) is water as shown in fig.

Let O be an object lying in the denser medium and the rays starting from it are as shown in fig. below,

                                                                             

A ray of light from O falling perpendicular to the interface XY passes without any deviation along AB. A second ray OA1, making some angle with the normal on interface will refract along A1B1 shown. Third ray OA3 is falling at a particular angle of incidence called critical angle (i2= C) at point A3 and the ray after striking point A3 bends towards the interface A3B3, in the case the angle of refraction is 90°.

                        Another ray OA4 falling at the interface XY at an angle greater than the critical angle (i3 > C), the rays after striking at point A4 will be reflected back in the medium (II) along A4B4 as shown in fig. This process is called Total internal Reflection (TIR).

Hence in short, we can conclude that when  a ray of light traveling from an optically denser medium to an optically rarer medium is incident at an angle greater than the critical angle for the pair of medium in contact, the ray is totally reflected back into denser medium. This process is called Total Internal Reflection.

Relation between refractive index and critical angle :

               ^Iµ_{II  = 1/sin C}

As is seen from fig., if the angle of incidence (i3 >C) greater than critical angle, the light ray is completely reflected back into the water (the denser medium).

Applications of Total Internal Reflection :

1. The Brilliance of Diamond : Refractive index of diamond is approximately 2∙45

⇒            Sin C = 1/µ = 1/2∙45 = 0∙408

⇒                  C = 1/sin (0∙408) = 24∙1°

i.e., critical angle for diamond is 24∙1. 

Diamond is cut in such a way that a ray of light enters the diamond at an angle greater than critical angle i.e., 24∙1°, hence light ray suffers multiple total internal reflections at different faces and remains within the diamond. This makes the diamond sparkle.

2. Total Internal Reflection in Prisms : 

For a glass prism,      µ = 1∙5

                          Sin C = 1/µ            

                                  = 1/1∙5 = 0∙667

                               C = 1/sin (0∙667) =42°

Thus, in a right angled isosceles prism, a ray of light incident at an angle of incidence 45° (i.e., greater than critical angle 42°), the light ray will be totally internally reflected.

                          

                          

                                       

3. Mirage :It is an optical illusion observed in deserts due to total internal reflection of light. Mirage disappoints a traveller in a sandy desert because a traveller on a look out for some supply of water gets feeling of presence of a pond of water as he sees the image of tree down below but did not get any water.
                    

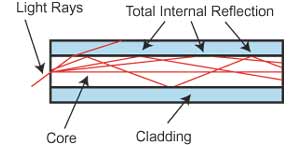
4. Optical Fibres : Optical fibers are made up of glass or plastic may be as thin as a human hair. Optical fibers have two parts (i) Core (ii) Cladding

(i) A Core is the central part or main part of an optical fibre. Diameter of core varies from 6µm to 250 µm. It is made from a pure glass of high refractive index (approximately 1⋅7)

(ii) Cladding: Core is surrounded by a cladding of glass or plastic of relatively small refractive index (approximately 1⋅5). Cladding is usually between 10 µm to 150 µm thick. The interface between core and cladding acts as a cylindrical mirror. A ray of light falling at this interface at an angle more than critical angle gets totally internally reflected.

                               

                       

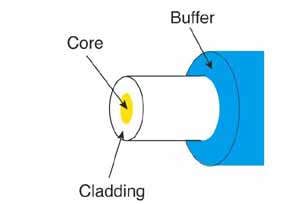
Optical fibres help in communication of signals in the form of light through many kilometers without loss in energy or signal. Optical fibers are also used in medical instruments like fibrescope to examine internal body cavities such as the stomach, galbladder etc.

5. Shinning of air bubble in water.


Practice Problem:

If refractive index of water and glass are 4/3 and 5/3 respectively and the light is going from glass  to water. Find the critical angle (C).
Solution:

             Given,            ^aµ_w =  4/3,    ^aµ_g =  5/3

                                 ^wµ_g  = ^aµ_{g /} ^aµ_w     

                                       =  5/3 X 3/4   

                                       = 5 /4  = 1⋅25
                                             
We know that,           ^wµ_g  =  1/Sin C

∴                      1/Sin C   = 1⋅25
⇒                        Sin C   = (1/1⋅25) = 0⋅8
                              C     =  (1/Sin) (0⋅8)
                              C      = 53⋅13° (Ans.)


Some Multiple choice Practice Problems :
1. The critical angle of light passing from glass to air is minimum for 
(a) red                               (b) green
(c) yellow                           (d)violet

2. A well cut diamond appears bright due to 
(a) it emits bright light 
(b) scattering of light
(c) total internal reflection
(d) it emits luminous particles

3.Total internal reflection will not take place, when light ray travels from
(a) glass to air
(b) glass to water
(c) water to air
(d) water to glass

4. µ = Sin C, where C is the angle of incidence in optically
(a) rarer medium              (b) denser medium
(c) air                              (d) transparent

5. Critical angle for a pair of media in contact is the angle of incidence in the denser medium corresponding to which angle of refraction in the rarer medium is
(a) 180°                          (b) 30°
(c) 45°                            (d) 90°

6.Condition for Total Internal Reflection is
(a) ∠i in denser medium should be greater than critical angle
(b) Light should travel from denser medium to rarer medium
(c)  ^Iµ_{II = 1/ Sin C
(d) All the above are true}

7. If the critical angle for total internal reflection from a medium to vacuum is 30°, then velocity of light in medium is
(a) 1⋅5 x 10⁸ m/s                   (b) 0⋅75 x 10⁸ m/s    

(c) 3 x 10⁸ m/s                      (d) 2 x 10⁸ m/s    

Answers :
1. d
2. c
3. d
4. b
5. d
6. d
7. c


coutesy:R.a.Banwat
thefoa.org
tutorvista.com
sa.ac.th